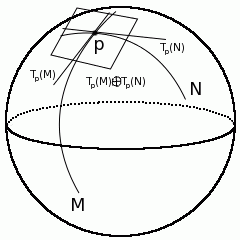
Geometry and topology are two distinct topics, in which the branch of geometry analyzes metric space and the study of topology investigates topological space. In Euclidean geometry, a set of elements existing within three dimensions has a metric space which is defined as the distance between two elements in the set. Conversely, topological space is a concept which considers Euclidean geometry and looks to generalize the structure of sets.
More specifically, in mathematics topological space is defined as a pair (X, T). In this pairing, X represents a set and T is a topology of a collection of subsets on X. This set also has a set of particular properties such as T needing to encompass both X and the empty set. It is critical to understand the definition of a topological space so that proofs can be completed to identify different topologies, such as discrete and indiscrete topologies.
Simply put topology aims to elucidate upon the qualitative elements of geometrical shapes and structures. Geometry analyzes shapes and structures in flat space, such as circles and polygons and investigates the properties of these structures. Whereas geometry is concerned with whether certain shapes may be congruent or not, topology considers different problems, such as whether these shapes are connected or separated.
Geometry and topology are two very important subjects in the discipline of mathematics. Furthermore, these topics extend into other mathematical areas such as combinatorics and algebraic geometry.
© BrainMass Inc. brainmass.com June 30, 2024, 2:06 am ad1c9bdddf